6.1.3.2
Fundamental frequency differential currents
The fundamental frequency differential current is a vectorial sum (sum of
fundamental frequency phasors) of the individual phase currents from the different
sides of the protected power transformer.
Before any differential current can be calculated, the power transformer phase shift,
and its transformation ratio, must be accounted for. Conversion of all currents to a
common reference is performed in two steps:
•
all current phasors are phase-shifted to (referred to) the phase-reference side,
(whenever possible the first winding with star connection)
•
all currents magnitudes are always referred to the first winding of the power
transformer (typically transformer high-voltage side)
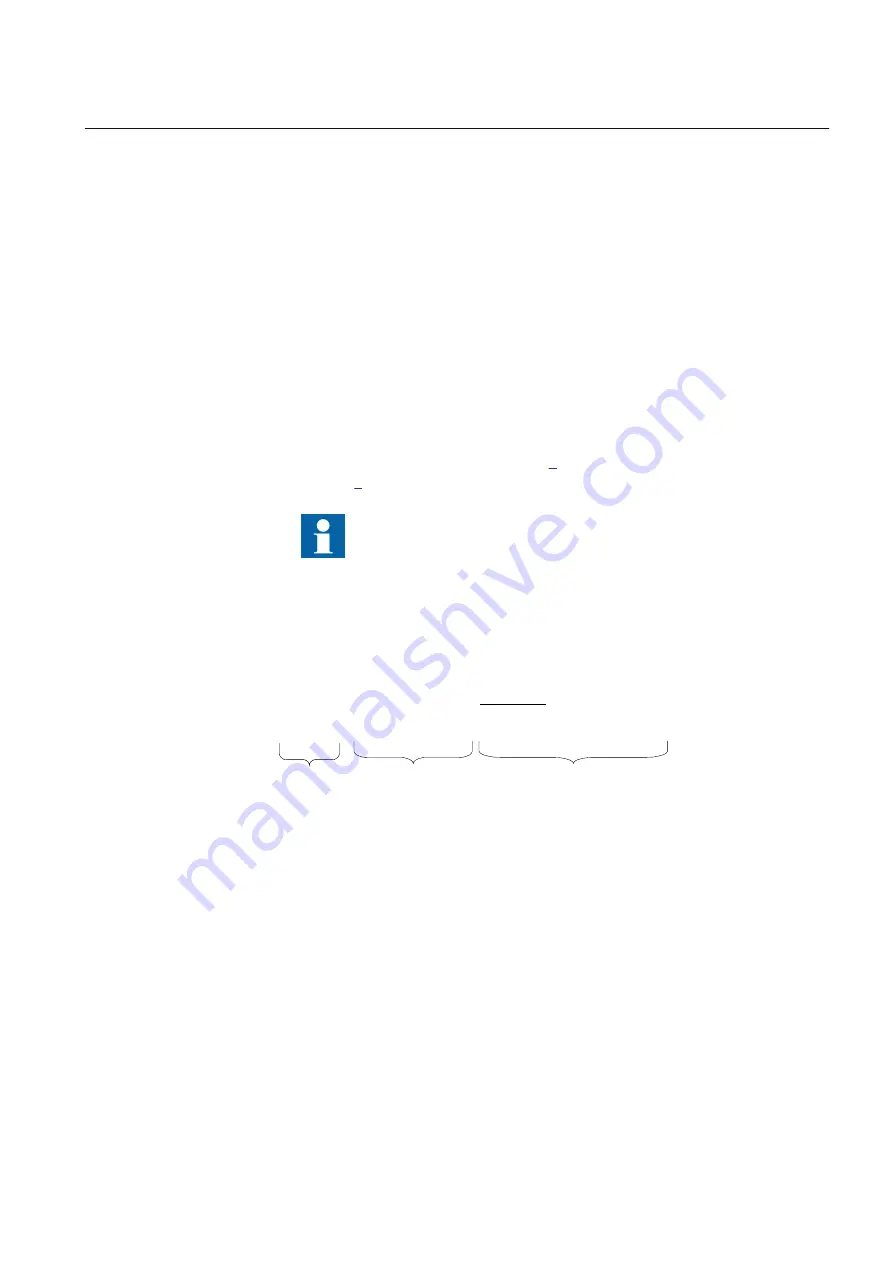
The two steps of conversion are made simultaneously on-line by the pre-programmed
coefficient matrices, as shown in equation
for a two-winding power transformer, and
for a three-winding power transformer.
These are the internal compensation algorithms within the differential
function. The protected power transformer data is always entered per
its nameplate. The Differential function will adapt nameplate data and
select proper reference windings.
1
1_ 1
1_ 2
_ 2
2
2 _ 1
2 _ 2
_ 1
3
3 _ 1
3 _ 2
IDL
IL
W
IL
W
Un W
IDL
A IL
W
B IL
W
Un W
IDL
IL
W
IL
W
é
ù
é
ù
é
ù
ê
ú
ê
ú
ê
ú
= ×
+
× ×
ê
ú
ê
ú
ê
ú
ê
ú
ê
ú
ê
ú
ë
û
ë
û
ë
û
1
2
3
EQUATION1880 V1 EN
(Equation 1)
where:
1.
is the resulting Differential Currents
2.
is the current contribution from the W1 side
3.
is the current contribution from the W2 side
1MRK 502 048-UEN A
Section 6
Differential protection
87
Technical manual
Summary of Contents for REG650 ANSI
Page 1: ...Relion 650 series Generator protection REG650 Technical manual ...
Page 2: ......
Page 36: ...30 ...
Page 42: ...36 ...
Page 50: ...44 ...
Page 64: ...58 ...
Page 86: ...80 ...
Page 262: ...256 ...
Page 300: ...294 ...
Page 438: ...432 ...
Page 476: ...470 ...
Page 592: ...586 ...
Page 664: ...658 ...
Page 678: ...672 ...
Page 726: ...720 ...
Page 727: ...721 ...
















































