=
C
C
Line
X
K
X
EQUATION1895 V1 EN-US
(Equation 100)
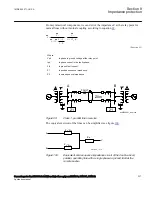
A typical 500 km long 500 kV line is considered with source impedance
1
0
=
SA
Z
EQUATION1896 V1 EN-US
(Equation 101)
~
E
A
Z
SA1
Power line
A
B
Seires
capacitor
Load
en06000585.vsd
IEC06000585 V1 EN-US
Figure 106:
A simple radial power system
en06000586_ansi.vsd
0
200
400
600
800
1000
1200
1400
1600
1800
100
200
300
400
500
P[MW]
V
[k
V
]
V
limit
P
0
P
3
0
P
5
0
P
7
0
ANSI06000586 V1 EN-US
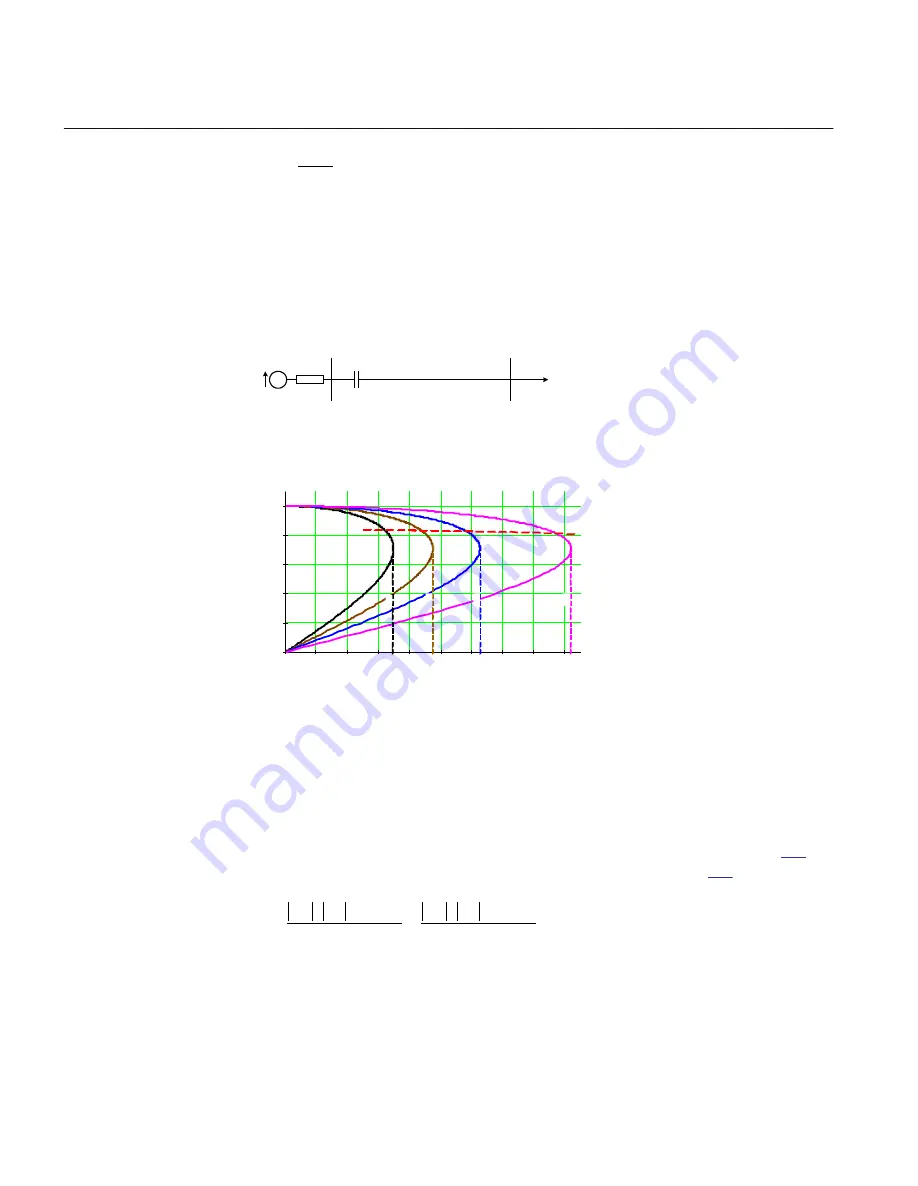
Figure 107:
Voltage profile for a simple radial power line with 0, 30, 50 and 70% of
compensation
8.3.3.2
Increase in power transfer
GUID-C9163D4E-CC2B-4645-B2AC-2C8A3FE3D337 v3
The increase in power transfer capability as a function of the degree of compensation
for a transmission line can be explained by studying the circuit shown in figure
The power transfer on the transmission line is given by the equation
( )
( )
(
)
A
B
A
B
Line
C
Line
C
V
V
sin
V
V
sin
P
X
X
X
1 K
d
d
×
×
×
×
=
=
-
× -
EQUATION1994-ANSI V1 EN-US
(Equation 102)
The compensation degree K
c
is defined as equation
Section 8
1MRK 502 071-UUS A
Impedance protection
246
Generator protection REG670 2.2 ANSI and Injection equipment REX060, REX061, REX062
Application manual
Summary of Contents for RELION 670 SERIES REG670
Page 1: ...RELION 670 SERIES Generator protection REG670 Version 2 2 ANSI Application manual ...
Page 2: ......
Page 44: ...38 ...
Page 66: ...60 ...
Page 102: ...96 ...
Page 200: ...194 ...
Page 442: ...436 ...
Page 486: ...480 ...
Page 508: ...502 ...
Page 514: ...508 ...
Page 524: ...518 ...
Page 658: ...652 ...
Page 736: ...730 ...
Page 774: ...768 ...
Page 828: ...822 ...
Page 829: ...823 ...