Armfield Instruction Manual
24
For the subsequent "square" area dF' the discharge is
dq' = Kdh' …. (6)
However, we can get only as much water into dF' as has passed through dF.
There is simply no other place from which the water could come. Nor is there
any other place where the water from dF could go. Therefore we have:
dq = dq' ........(7)
which, from equations (5) and (6) gives:
dh = dh' ........(8)
This is a very important result. It implies that the potential drop between two
adjacent pairs of equipotential lines is the same if they share the same pair of
adjacent flow lines and enclose an area similar to a square.
If a pair of flow lines is given, there is only one way to divide the strip between
them into a sequence of "squares" (ie. tetragonals) whose four corners form
right angles and whose mean distances between opposite faces are alike.
Therefore, if we succeed in dividing the strip between the two flow lines into a
sequence of such "squares", we can use their number n for calculating the
potential drop dh between two successive equipotential lines. Since all the
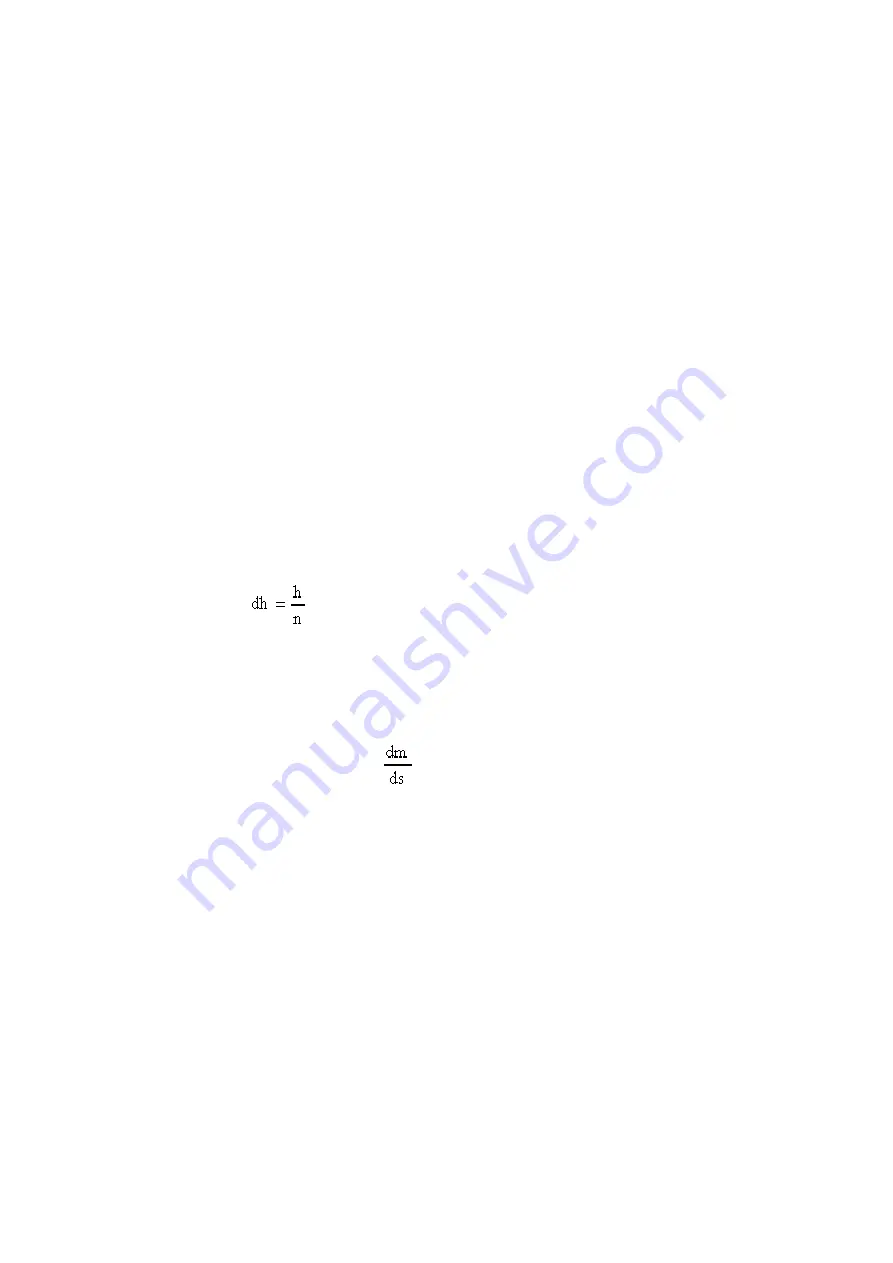
values of dh must be the same according to equation (8) we have:
......... (9)
Knowing dh we can finally determine from equation (5) the discharge (per unit
length) through the area between two flow lines.
Although it is advantageous to have a "square" flow net, it is also possible to
determine dh from a rectangular net if all the rectangles between two flow
lines have the same ratio
. Denoting this ratio by c, equations (5) and
(6) become respectively.
dq = K c dh .........(10)
dq' = K c dh ..........(11)
which again means that equation (8) holds and we can obtain dh from
equation (9) where n is the number of rectangles between the two flow lines.
Discharge dq is then obtained from equation (10).
If, for some reason, we do not have a flow net consisting of squares or
geometrically similar rectangles, we would have to measure the actual heads
at all equipotential lines in order to obtain the potential drops between each.
















































