20060301
15-3-3
Compound Interest
Calculation Formula
s
S
PV, PMT, FV,
n
I
%
&
0
I
% = 0
PV
= – (
PMT
s
n
+
FV
)
FV
= – (
PMT
s
n
+
PV
)
PV
=
–
×
PMT
–
×
FV
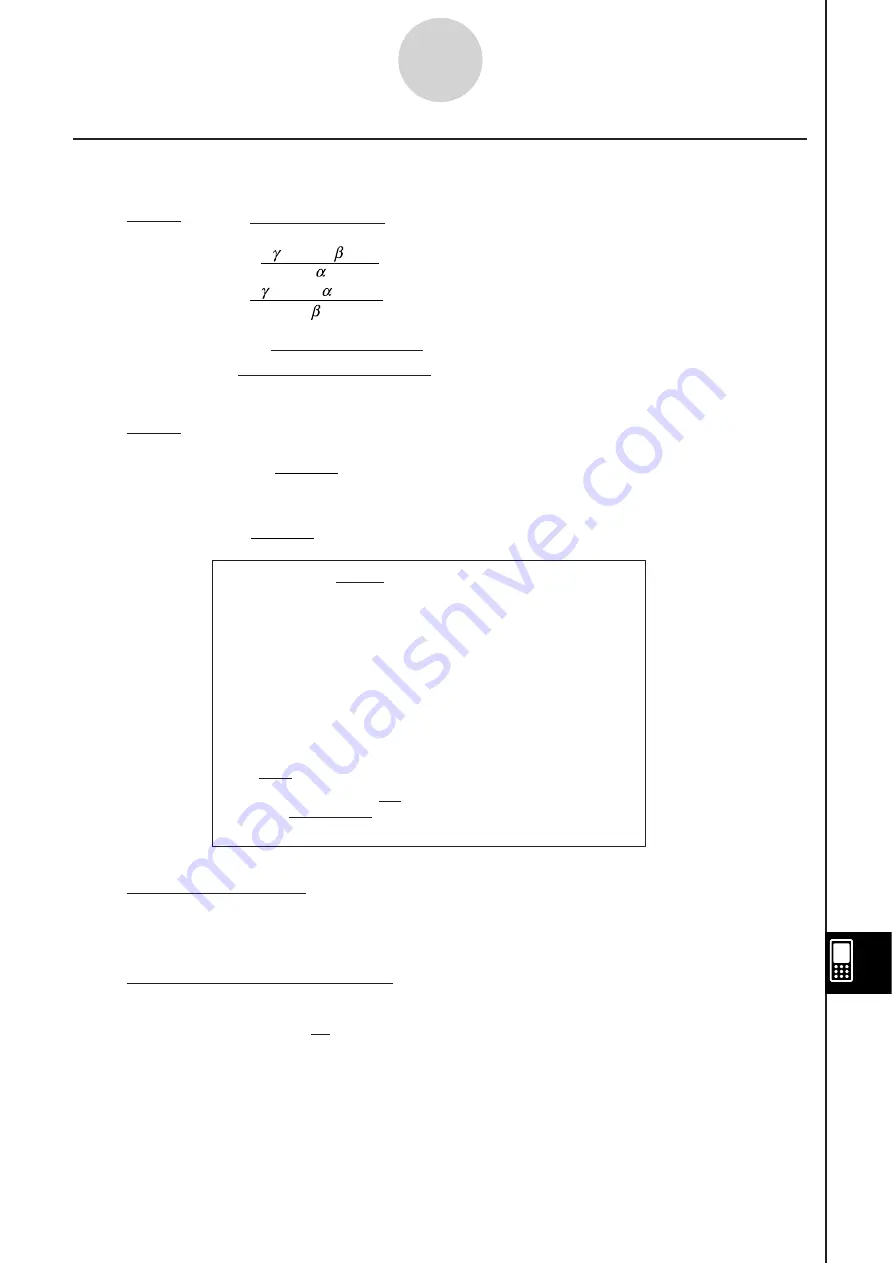
β
γ
α
PMT
=
–
s
PV
–
s
FV
FV
=
–
s
PV
–
s
PMT
n
=
log
(1+
iS
)
×
PMT
–
FV
×
i
(1+
iS
)
×
PMT
+
PV
×
i
{
}
log (1+
i
)
PMT
= –
n
PV
+
FV
n
=
PMT
PV
+
FV
–
S
I
%
i
(effective interest rate)
i
(effective interest rate) is calculated using Newton’s Method.
G
s
PV +
A
s
PMT +
B
s
FV = 0
To
I
% from
i
(effective interest rate)
Tip
• Interest (
I
%) calculations are performed using Newton’s Method, which produces approximate
values whose precision can be affected by various calculation conditions. Interest calculation
results produced by this application should be used keeping the above in mind, or results should
be confirmed separately.
= (1+
i
×
S
)
×
i
1
–
β
α
i
=
100
I
%
{
I
%
(1+
)
–1
C
/
Y
P
/
Y
100
×
[
C
/
Y
]
............................... (
P/Y
=
C/Y
= 1)
(Other than those above)
0 ............................ Payment : End (Format tab)
1 ............................ Payment : Begin (Format tab)
{
S
=
.....
1 ........................... Off (Format tab)
(1+
i
)
Frac
(
n
)
........... CI (Format tab)
1+
i
×
Frac
(
n
) ....... SI (Format tab)
γ
=
{
(1+
i
)
−
n
................. Off (Format tab)
(1+
i
)
−Intg
(
n
)
............ CI
or SI
(Format tab)
{
ß
=
{ }
×
C
/
Y
×
100...
I
%
=
(1+
i
)
–1
P
/
Y
C
/
Y
(Other than those above)
i
×
100 .................................
(
P
/
Y = C
/
Y =
1)
{
Summary of Contents for ClassPad 330
Page 11: ...20060301 20090601 ClassPad 330 ClassPad OS Version 3 04 ...
Page 277: ...20060301 3 3 10 Storing Functions 3 Tap AND Plot OR Plot ...
Page 779: ...20090601 S Graph Line Clustered C S Graph Line Stacked E 13 9 2 Graphing ...
Page 780: ...20090601 S Graph Line 100 Stacked F S Graph Column Clustered G 13 9 3 Graphing ...
Page 781: ...20090601 S Graph Column Stacked I S Graph Column 100 Stacked J 13 9 4 Graphing ...
Page 782: ...20090601 S Graph Bar Clustered K S Graph Bar Stacked 9 13 9 5 Graphing ...
















































