E-26
•
Example:
Atmospheric Pressure vs. Temperature
Perform linear regression to de-
termine the regression formula
terms and correlation coefficient
for the data nearby. Next, use
the regression formula to esti-
mate atmospheric pressure at
–5
°
C and temperature at 1000
hPa. Finally, calculate the coeffi-
cient of determination (
r
2
) and
sample covariance
.
In the REG Mode:
1
(Lin)
A
B
1
(Scl)
=
(Stat clear)
10
P
1003
S
Each time you press
S
to register your input,
the number of data input up to that point is
indicated on the display (
n
value).
15
P
1005
S
20
P
1010
S
25
P
1011
S
30
P
1014
S
Regression Coefficient A =
997.4
A
X
r
r
1
=
Regression Coefficient B =
0.56
A
X
r
r
2
=
Correlation Coefficient
r
=
0.982607368
A
X
r
r
3
=
Atmospheric Pressure at
5
°
C =
994.6
E
D
5
F
A
X
r
r
r
2
=
Temperature at 1000 hPa =
4.642857143
1000
A
X
r
r
r
1
=
Coefficient of Determination =
0.965517241
A
X
r
r
3
K
=
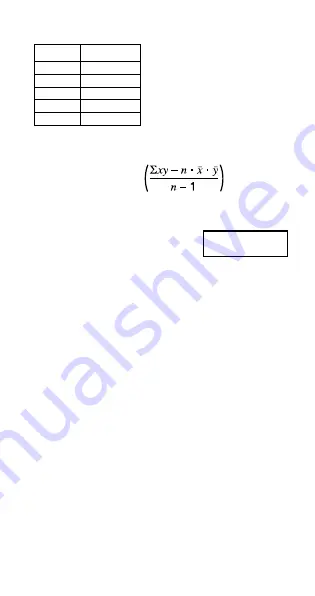
Sample Covariance =
35
E
A
U
r
3
,
A
U
3
-
A
X
1
-
A
X
r
1
F
\
E
A
U
3
,
1
F
=
Temperature
Atmospheric
Pressure
10
°
C
1003 hPa
15
°
C
1005 hPa
20
°
C
1010 hPa
25
°
C
1011 hPa
30
°
C
1014 hPa
1.
n=
REG













































