EE Pro for TI-89, 92 Plus
Equations - Motors and Generators
128
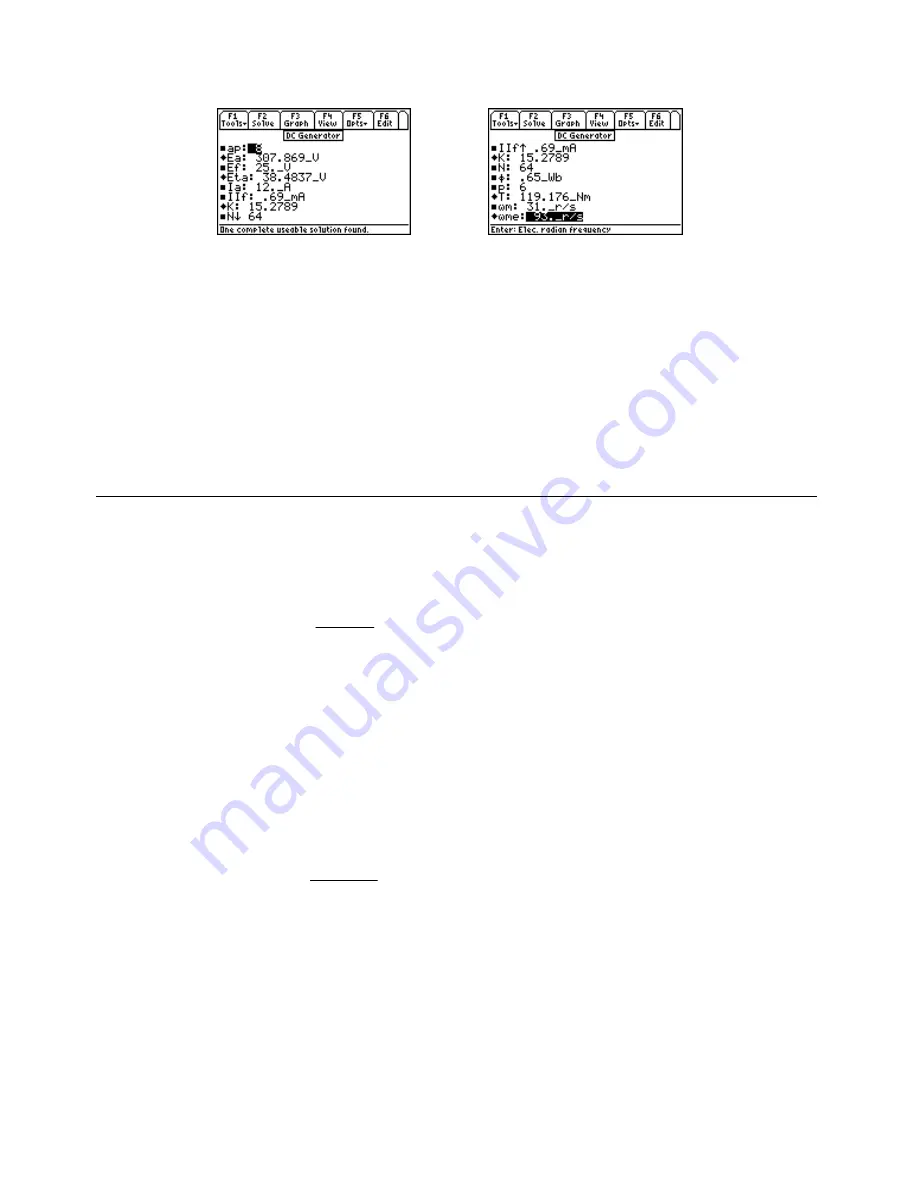
Display: Upper Half
Display: Lower Half
Solution -
Choose the first six equations. Select these by highlighting each equation and pressing
¸
. Press
„
to display the input screen, enter all the known variables and press
„
to solve the
selected equation set. The computed results are shown in the screen displays above.
-PQYP8CTKCDNGU
CR
'H
A8
+C
A#
++H
AO#
0
φ
A9D
R
ω
O
ATU
%QORWVGF4GUWNVU
'C
A8
'VC
A8
-
6
A0O
ω
OG
ATU
31.3 Separately-Excited DC Generator
The equations in this section describe the properties of a separately excited DC generator. The first equation
computes the field current IIf in terms of field voltage Vfs, external shunt resistance re, and field coil resistance Rf.
The next equation evaluates armature induced voltage Ea as a function of machine constant K, mechanical radian
frequency
ω
m, and flux
φ
.
IIf
Vfs
Rf
=
+
Re
Eq. 31.3.1
Ea
K
m
= ⋅
⋅
ω φ
Eq. 31.3.2
The third and fourth equations are alternate forms of expressing terminal voltage Vt in terms of load current IL, load
resistance Rl, armature resistance Ra.
Vt
IL Rl
=
⋅
Eq. 31.3.3
Vt
Ea
Ra IL
=
−
⋅
Eq. 31.3.4
Finally the armature current IL in terms of K,
φ
,
ω
m, Ra and Rl.
IL
K
m
Ra
Rl
= ⋅ ⋅
+
φ ω
Eq. 31.3.5
Example 31.3 -
A DC generator with a machine constant of 3.8 is driving a load of 46 k
Ω
and rotates at a speed
of 31 rad/s. The magnetic flux is 1.6 Wb. The field is driven by a 24 V source. The field coil resistance is
10
Ω
. The armature resistance is 13
Ω
in series with an external resistance of 55
Ω
. Find the field current, armature
induced voltage and the terminal voltage.
















































