A-4
MIGII Digital Protection for Electrical Machines
GEK-113188A
A.3 ALGORITHMS
APPENDIX A
A
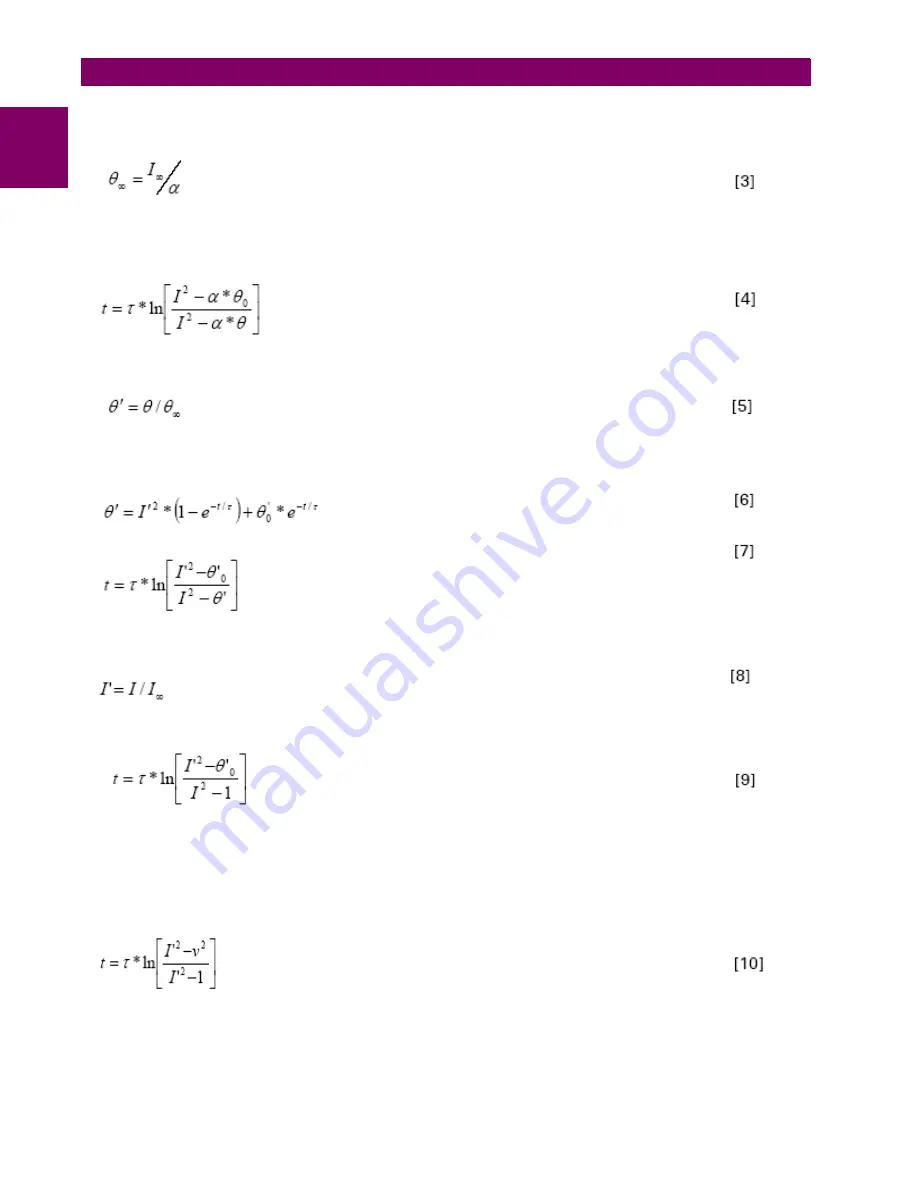
Obviously, the derived equation describes the temperature evolution for both, a heating process and a cooling process.
The final temperature value
θ
∞
, for a permanent current I
∞
, will be (according to [2]):
In equation [2], solving for time, you get:
Introducing the following variable change:
that implies to refer temperatures to the steady state value, equations [2] and [4] can be written as :
where I’ represents the current value in per unit, based on the permanent current, this is:
To compute the tripping time, substitute in [7], with
θ
’ = 1, and you get:
It is necessary that I > 1.
Equation [9], can also be written as a function of current, in p.u., if it has been maintained permanently (in
other case, it is necessary to compute the equivalent current), that is represented by the letter “
v
”:
Equation [10], represents the basic tripping algorithm for a thermal image relay, that for a given
τ
and I
∞
, can be drawn, in
general using a logarithm plane, using “v” as the parameter, as shown in Figure A–1: and Figure A–2:
Summary of Contents for Multilin MIGII
Page 2: ......













































