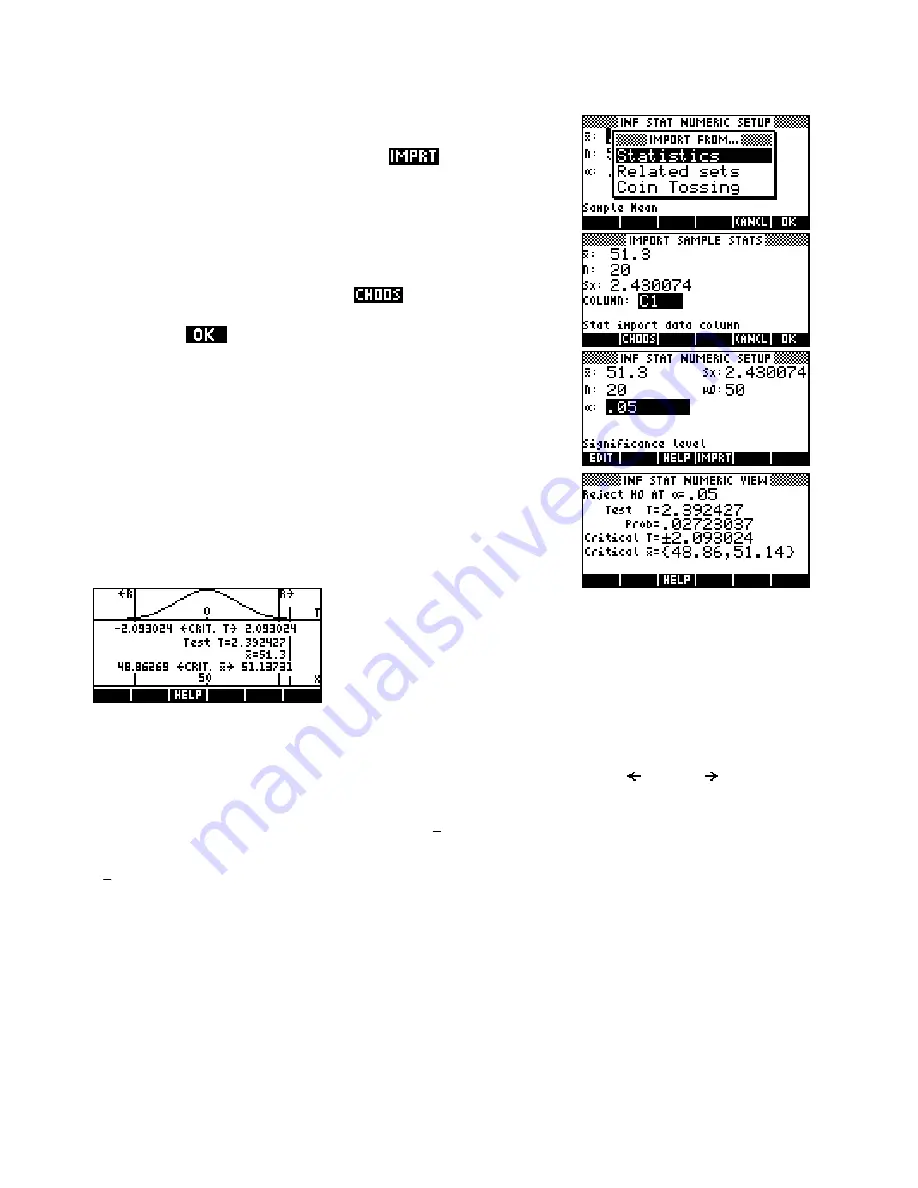
Change now to the
NUM SETUP
view to enter the required values.
Rather than entering them by hand, press the
key. If you have
more than one copy of the Statistics aplet (under other names) then you
will be presented with a list of aplets from which to choose.
Once you have chosen the aplet, you need to nominate the column from
which to import the data. The default is column
C1
, which is what we
want in this case, but you can press the
key to select from a list
of any other columns which contain data. When you have the correct
column, press
.
Enter the population mean
µ
0
= 50, and check that the test level is
correct at 0.05 (5%).
If you now change to the
NUM
view you will see the inferential data in
numeric form. The test Student-t value is given as 2.392 and the
probability of obtaining such a value as 0.0272. The critical t values for
this test level of 5% are given as
±
2.093 and the critical boundaries for
the sample mean as 48.86 and 51.14.
The information can be seen in a more visual form if you change to the
PLOT
view as can be seen left. The upper normal curve shows the
critical range for the t values, together with a short vertical line showing
the position of the Test T value. The lower horizontal line shows the
equivalent critical sample mean range.
The test value for the Student-t and the sample mean are also listed in the middle of the screen. The regions
for rejection of the null hypotheses are shown at the very top of the screen by the ‘ R’ and ‘R ’.
We assume, by statistical theory, that the distance
(
x
−
µ
)
is normally distributed. If the null hypothesis is true
then the mean of this new distribution should be zero. Our result is indicating that our particular value of
(
x
−
µ
)
is of such a size that if the null hypothesis is true then the probability of it appearing by chance is less
than 5%.
In this case, whether you work from the
PLOT
view or the
NUM
view, it is clear from the evidence that the
null hypothesis should be rejected, with less than a 5% chance of this rejection being incorrect. The alternate
hypothesis that the mean is not 50 should be accepted. Of course in this case, despite some boxes
containing less than 50 matches, the actual mean seems to be above 50 so we can hardly condemn the
company for putting more matches in the boxes than they need to!
142













































