Method 3 - Using the
POLYROOT
function
The advantage of this is that it can be done in the
HOME
view and is
quick and easy. It also has the advantage that it returns complex roots
as well. See page 84 for a method of copying the results to a matrix so
as to gain easier access to them. This method is highly recommended
for polynomials in general.
F
F
i
i
n
n
d
d
i
i
n
n
g
g
c
c
o
o
m
m
p
p
l
l
e
e
x
x
s
s
o
o
l
l
u
u
t
t
i
i
o
o
n
n
s
s
t
t
o
o
a
a
c
c
o
o
m
m
p
p
l
l
e
e
x
x
e
e
q
q
u
u
a
a
t
t
i
i
o
o
n
n
( )
=
z
2
+ +
1
.
Find the roots of the complex equation
f z
z
Method 1 - Using the QUAD function
Since this is a quadratic it can be done with the
QUAD
formula
mentioned in example 1., since it is capable of giving complex results.
This is shown right, rounded to 4 dec. pts. It’s up to you of course to
realize that (0,1.7321) is
just that portion and square it. The ‘
S1
’ means ± . The only real
advantage of this method is that it gives the answer in the same format
as the quadratic formula and this may be of use.
3
i
but if you don’t recognize it then
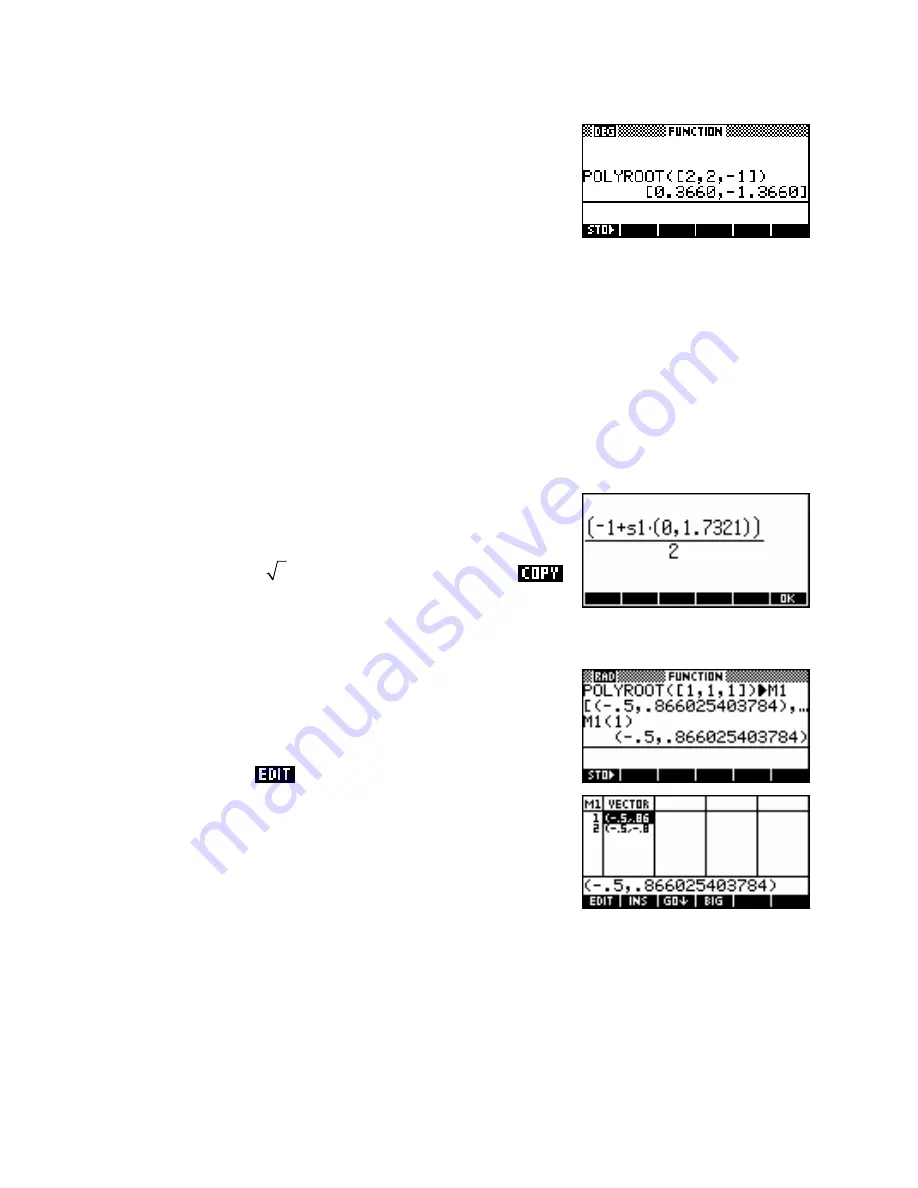
Method 2 - Using POLYROOT
An alternative method is to use the
POLYROOT
function and store the
results to a matrix. This offers the advantage of being able to examine
the result more easily by
ing the matrix, and also of being able to
access each root by referring to the matrix elements in a calculation (eg
M1(1), M1(2) etc.). See page 84 for more details on this.
The disadvantage of this method is that for higher degree polynomials
with a mixture of real and complex roots, all roots are shown in complex
form. This can make the real roots harder to isolate and use. However,
it is still the best method.
Method 3 - Using the CAS on the hp 40gs
See page 309 for an example of finding roots of real and complex polynomials using the CAS on the
hp 40gs.
299




































