Page 9-18
Thus the angle between vectors
r
and
F
is
θ
= 41.038
o
. RPN mode, we can
use:
[3,-5,4]
`
[2,5,-6]
`
CROSS ABS
[3,-5,4]
`
ABS
[2,5,-6]
`
ABS
* /
ASIN
NUM
Equation of a plane in space
Given a point in space P
0
(x
0
,y
0
,z
0
) and a vector
N
= N
x
i
+N
y
j
+N
z
k
normal to a
plane containing point P
0
, the problem is to find the equation of the plane.
We can form a vector starting at point P
0
and ending at point P(x,y,z), a
generic point in the plane. Thus, this vector
r
= P
0
P = (x-x
0
)
i
+ (y-y
0
)
j
+ (z-z
0
)
k
,
is perpendicular to the normal vector
N
, since
r
is contained entirely in the
plane. We learned that for two normal vectors
N
and
r
,
N
•
r
=0. Thus, we
can use this result to determine the equation of the plane.
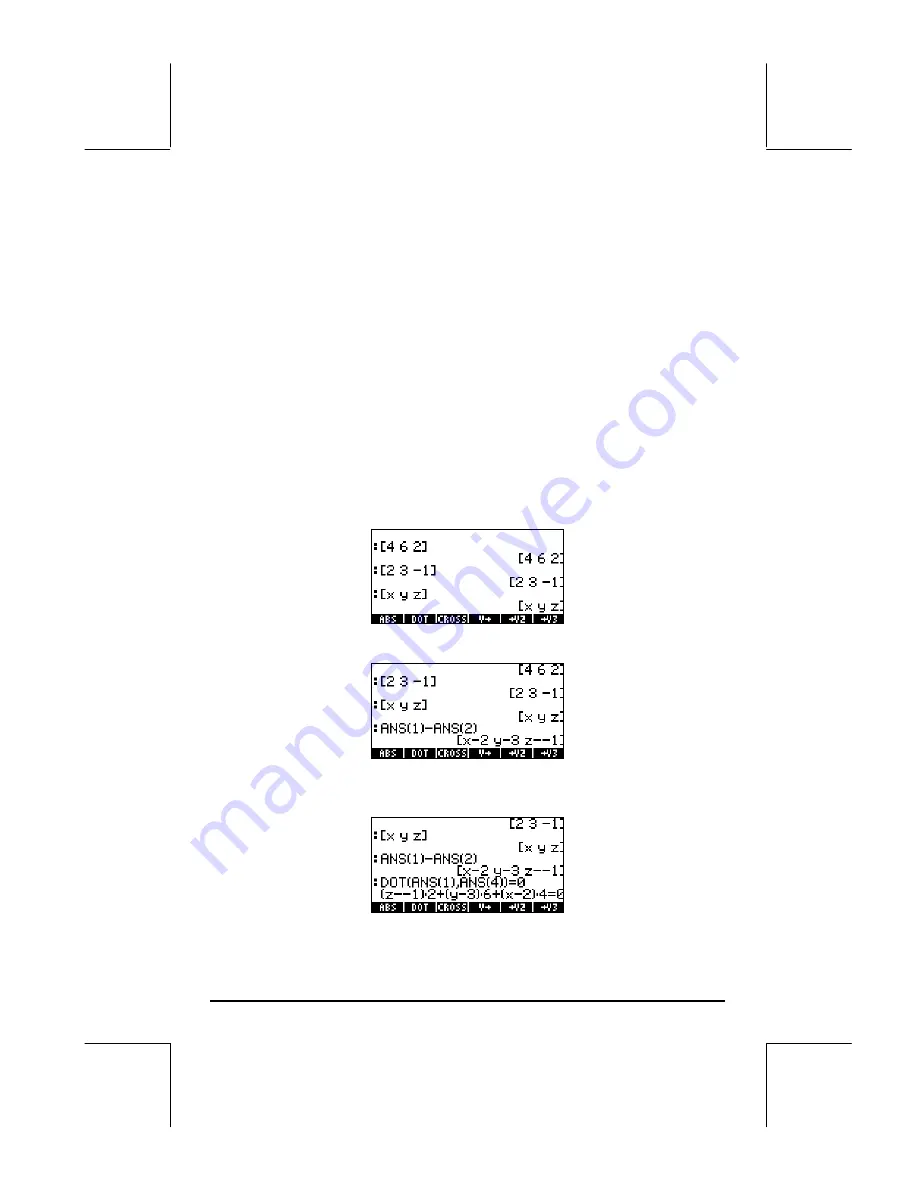
To illustrate the use of this approach, consider the point P
0
(2,3,-1) and the
normal vector
N
= 4
i
+6
j
+2
k
, we can enter vector
N
and point P
0
as two
vectors, as shown below. We also enter the vector [x,y,z] last:
Next, we calculate vector P
0
P =
r
as ANS(1) – ANS(2), i.e.,
Finally, we take the dot product of ANS(1) and ANS(4) and make it equal to
zero to complete the operation
N
•
r
=0:
















































