Page 11-29
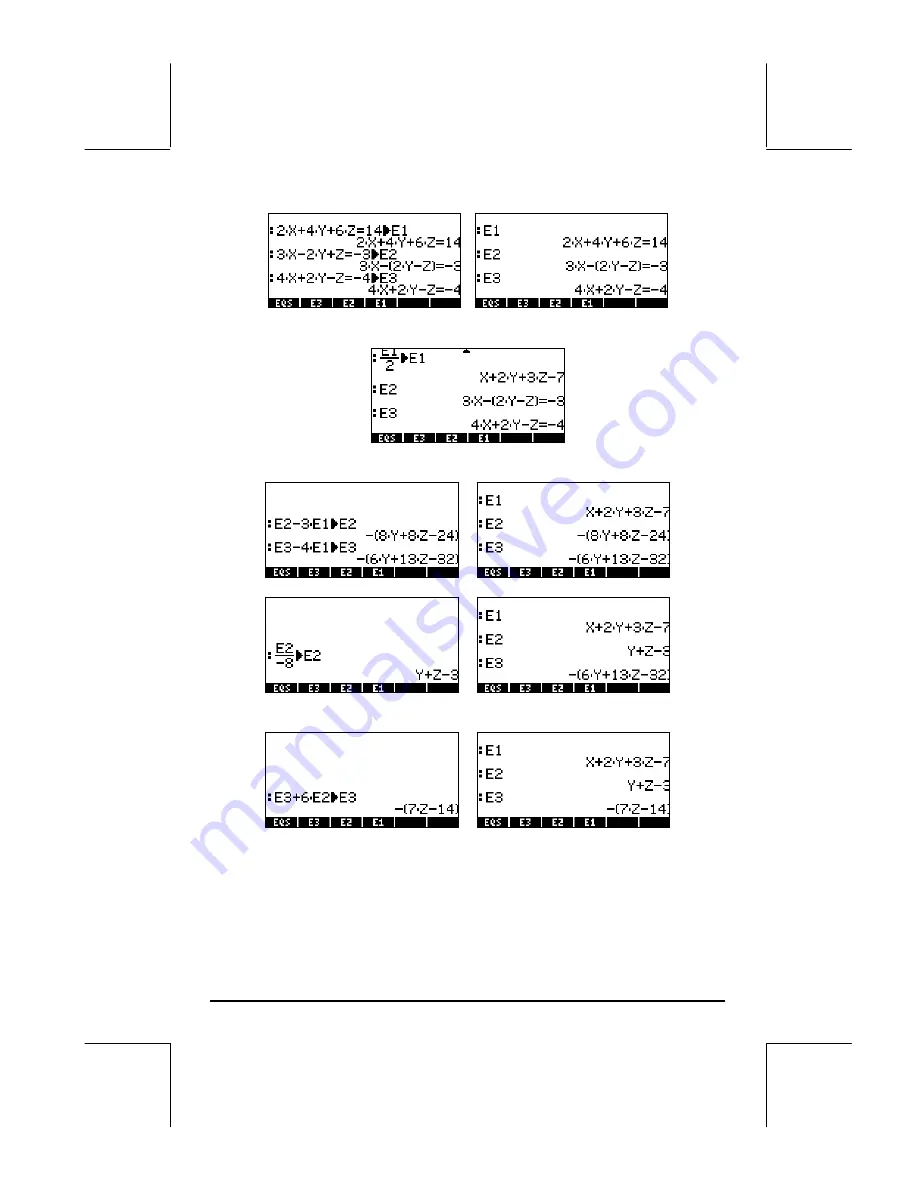
To start the process of forward elimination, we divide the first equation (E1) by
2, and store it in E1, and show the three equations again to produce:
Next, we replace the second equation E2 by (equation 2 – 3
×
equation 1, i.e.,
E1-3
×
E2), and the third by (equation 3 – 4
×
equation 1), to get
Next, divide the second equation by –8, to get
Next, replace the third equation, E3, with (equation 3 + 6
×
equation 2, i.e.,
E2+6
×
E3), to get
Notice that when we perform a linear combination of equations the calculator
modifies the result to an expression on the left-hand side of the equal sign, i.e.,
an expression = 0. Thus, the last set of equations is interpreted to be the
following equivalent set of equations:
X +2Y+3Z = 7,
















































