Page 16-30
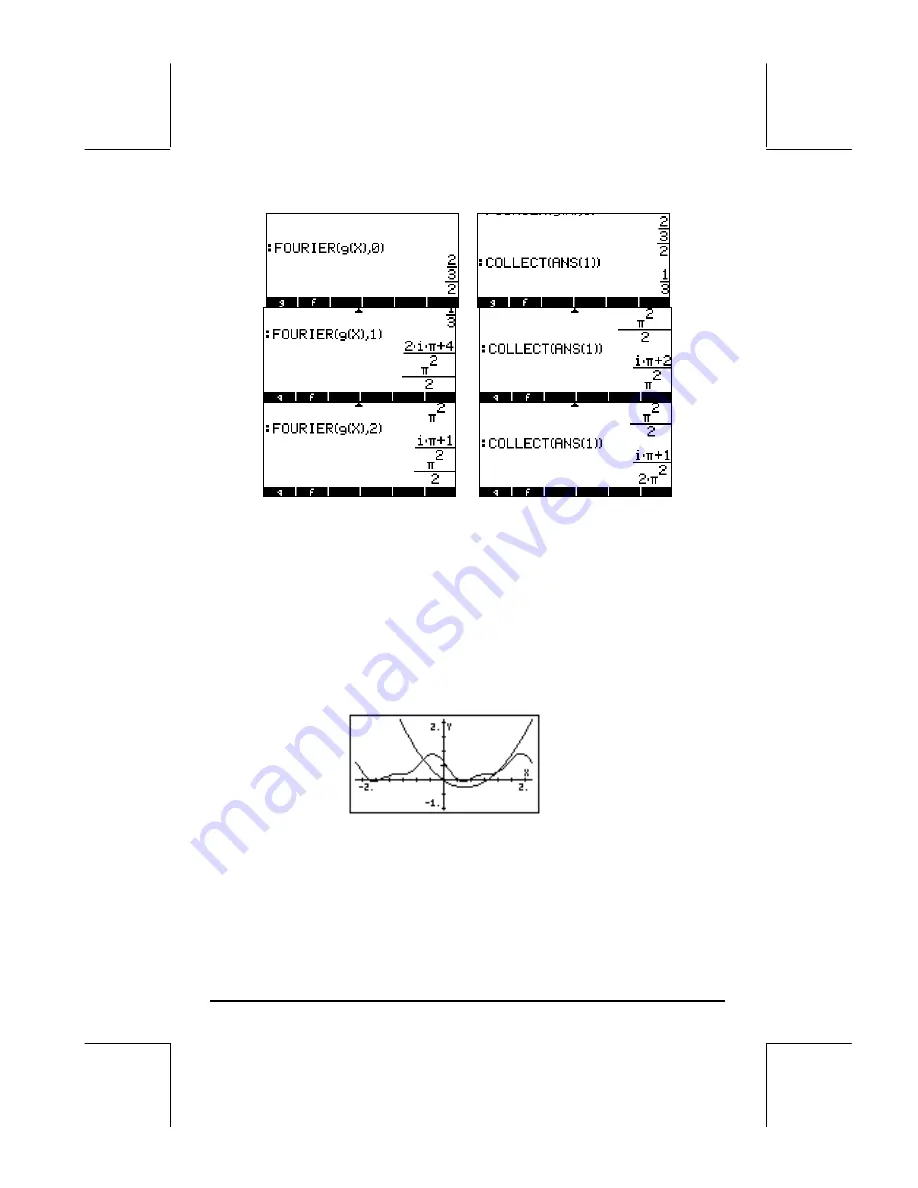
Thus, c
0
= 1/3, c
1
= (
π⋅
i+2)/
π
2
, c
2
= (
π⋅
i+1)/(2
π
2
).
The Fourier series with three elements will be written as
g(t)
≈
Re[(1/3) + (
π⋅
i+2)/
π
2
⋅
exp(i
⋅π⋅
t)+ (
π⋅
i+1)/(2
π
2
)
⋅
exp(2
⋅
i
⋅π⋅
t)].
A plot of the shifted function g(t) and the Fourier series fitting follows:
The fitting is somewhat acceptable for 0<t<2, although not as good as in the
previous example.















































