30
SYSTEM
If measurements of the transmission coefficient,
τ
, are made at several elevation angles, a
simple method from Lang (1987) can be used. The measurements of
τ
are used to compute
Equation 20
τ
=
Θ
Θ
y
cos ln
These are regressed on
Θ
(in radians), giving a slope (
B
) and intercept (
A
). LAI is given by:
Equation 21
=
+
L
A B
2(
)
An approximate value for
χ
is
Equation 22
χ
=
−
B
L
exp(
/ 0.4 )
EXAMPLE PROBLEM 3
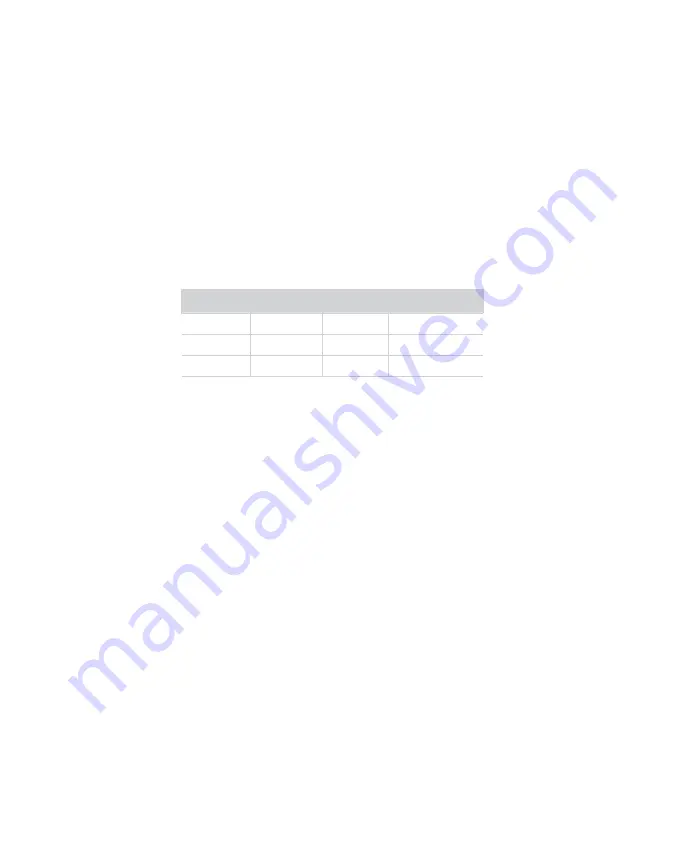
Readings were obtained as given in the following table:
Θ
-deg
Θ
-rad
τ
–cos
Θ
ln
τ
35
0.61
0.21
1.28
41
0.72
0.18
1.29
55
0.96
0.10
1.32
Linear regression gives the following values for each variable:
A
= 1.21
B
= 0.12
L
= 2(1.21 + 0.12) = 2.64
χ
= exp(-0.12 / 0.4 × 2.64) = 0.9
A more precise method for finding
χ
is as follows. Find values for
χ
and
L
which minimize:
τ
= Σ
+
F
K L
(ln
)
i
i
2
subject to the constraint,
χ
> 0, where
τ
i
are transmission coefficients measured at several
zenith angles
τ
i
and the
K
i
are the extinction coefficients for the corresponding angles.
3.4.5 CORRECTION OF PAR FOR SUN ANGLE
Canopy transmission (
τ
) measured at one zenith angle can be used to predict transmission
or radiation interception for other zenith angles.
EXAMPLE PROBLEM 4
A measurement might be made at
Θ
= 32° from which cover (1 - transmission at
Θ
= 0) is to
be calculated. From
: