Functions
6-47
7SA6 Manual
C53000-G1176-C156-2
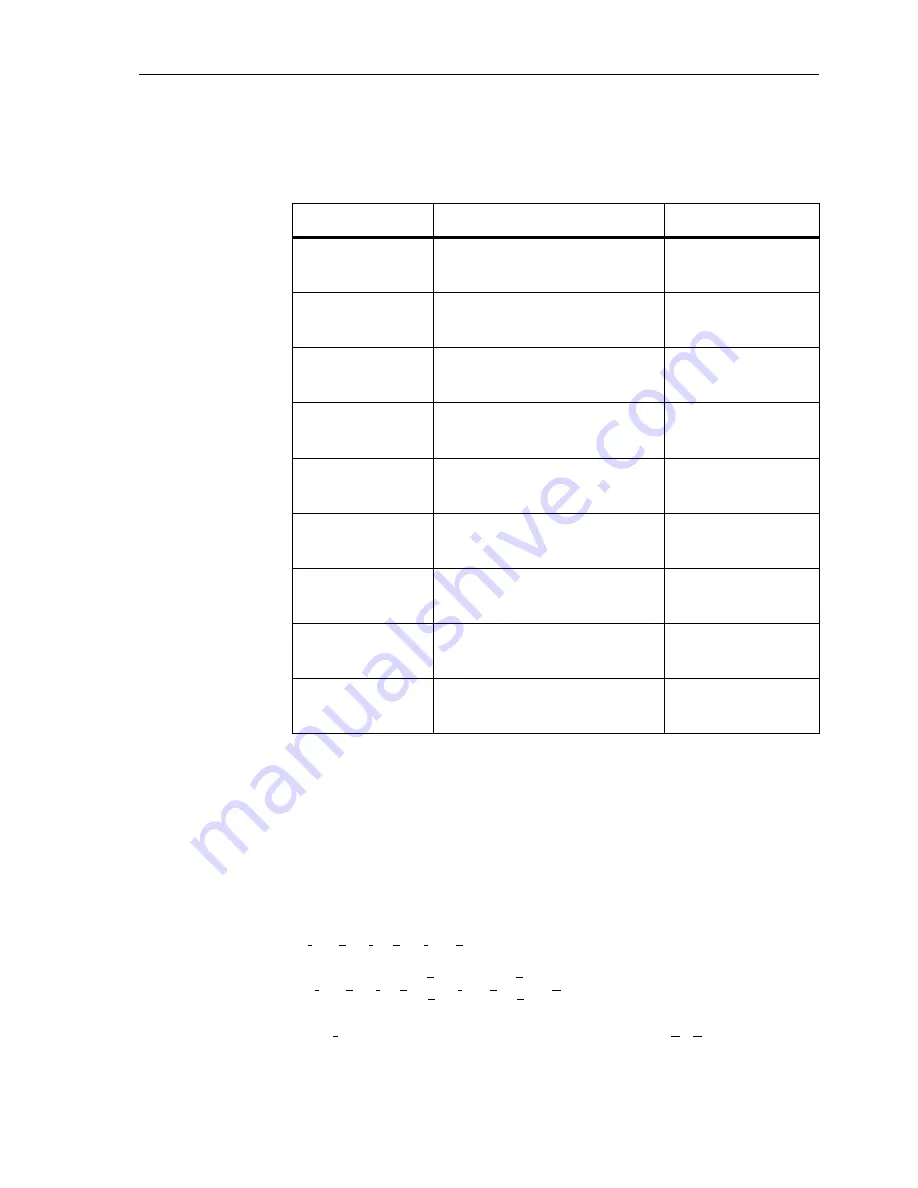
Table 6-8 lists all measured values used for the distance measuring in isolated or res-
onant-earthed systems.
Measured Value
Correction for
Parallel Lines
(optional)
During earth faults on parallel lines, the impedance values calculated by means of the
loop equations are influenced by the coupling of the earth impedance of the two con-
ductor systems (Figure 6-27). Unless special measures are employed, this results in
measuring errors in the result of the impedance computation. A parallel line compen-
sation may therefore be activated. In this manner the earth current of the parallel line
is taken into consideration by the line equation and thereby allows for compensation
of the coupling influence. The earth current of the parallel line must be connected to
the device for this purpose. The loop equation is then modified as shown below, refer
also to Figure 6-24
where
I
EP
is the earth current of the parallel line and the ratio Z
M
/Z
L
is a constant line
parameter, resulting from the geometry of the double circuit line and the nature of the
Table 6-8
Evaluation of measured loops for a multiple pick-up in non-earthed systems
Fault detection
Loops
Evaluated
Loop(s)
Setting
Parameter
1221
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L1–E
L3–E
L3–E
PHASE PREF.2phe
=
L3 (L1) acyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L1–E
L3–E
L1–E
PHASE PREF.2phe
=
L1 (L3) acyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L2–E
L2–E
L1–E
PHASE PREF.2phe
=
L2 (L1) acyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L1–E
L2–E
L1–E
PHASE PREF.2phe
=
L1 (L2) acyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L2–E
L3–E
L3–E
PHASE PREF.2phe
=
L3 (L2) acyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L2–E
L2–E
L3–E
PHASE PREF.2phe
=
L2 (L3) acyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L1–E
L2–E
L3–E
PHASE PREF.2phe
=
L3 (L1) cyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L2–E
L3–E
L1–E
PHASE PREF.2phe
=
L1 (L3) cyclic
L1–E, L2–E, (L1–L2)
L2–E, L3–E, (L2–L3)
L1–E, L3–E, (L3–L1)
L1–E, L2–E
L2–E, L3–E
L3–E, L1–E
PHASE PREF.2phe
=
All loops
I
L3
Z
L
I
E
Z
E
I
EP
Z
M
⋅
U
=
L3–E
–
⋅
–
⋅
I
L3
Z
L
I
E
Z
L
Z
E
Z
L
-------
I
EP
Z
L
Z
M
Z
L
-------
⋅
⋅
–
U
L3–E
=
⋅
⋅
–
⋅
Summary of Contents for siprotec 7SA6
Page 2: ...Siemens Aktiengesellschaft Book No C53000 G1176 C156 2 ...
Page 18: ...xviii 7SA6 Manual C53000 G1176 C156 2 ...
Page 32: ...Introduction 1 14 7SA6 Manual C53000 G1176 C156 2 ...
Page 82: ...Hardware and Connections 2 50 7SA6 Manual C53000 G1176 C156 2 ...
Page 119: ...SIPROTEC 4 Devices 4 25 7SA6 Manual C53000 G1176 C156 2 Figure 4 20 CFC Logic example ...
Page 190: ...Configuration 5 62 7SA6 Manual C53000 G1176 C156 2 ...
Page 652: ...Installation and Commissioning 8 78 7SA6 Manual C53000 G1176 C156 2 ...
Page 724: ...Technical Data 10 56 7SA6 Manual C53000 G1176 C156 ...
Page 800: ...Appendix A 76 7SA6 Manual C53000 G1176 C156 2 ...
Page 866: ...Appendix B 66 7SA6 Manual C53000 G1176 C156 2 ...
















































