PQM-700 Operating Manual
40
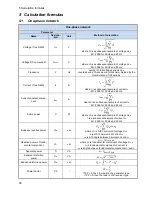
RMS value of positive
current sequence
I
1
A
𝐼
1
=
1
3
(𝐼
𝐴1
+ 𝑎𝐼
𝐵1
+ 𝑎
2
𝐼
𝐶1
)
𝐼
1
= 𝑚𝑎𝑔(𝐼
1
)
where
I
A1
,
I
B1
,
I
C1
are vectors of fundamental current
components
I
A
,
I
B
,
I
C
Operator
mag()
indicates vector module
RMS value of negative
current sequence
I
2
A
𝐼
2
=
1
3
(𝐼
𝐴1
+ 𝑎
2
𝐼
𝐵1
+ 𝑎𝐼
𝐶1
)
𝐼
2
= 𝑚𝑎𝑔(𝐼
2
)
where
I
A1
,
I
B1
,
I
C1
are vectors of fundamental compo-
nents for phase voltages
I
A
,
I
B
,
I
C
Operator
mag()
indicates vector module
Current unbalance factor
for zero sequence
i
0
%
𝑖
0
=
𝐼
0
𝐼
1
∙ 100%
Current unbalance factor
for negative sequence
i
2
%
𝑖
2
=
𝐼
2
𝐼
1
∙ 100%
5.4
3-phase wye and delta network without neutral conductor
3-phase wye and delta network without neutral conductor
(Parameters: RMS voltage and current, DC components of voltage and current, THD, flicker are calculated as for 1-phase circuits;
instead of the phase voltages, phase-to-phase voltages are used. Symmetrical components and unbalance factors are calculated
as in 3-phase 4-wire systems.)
Parameter
Method of calculation
Name
Designa-
tion
Unit
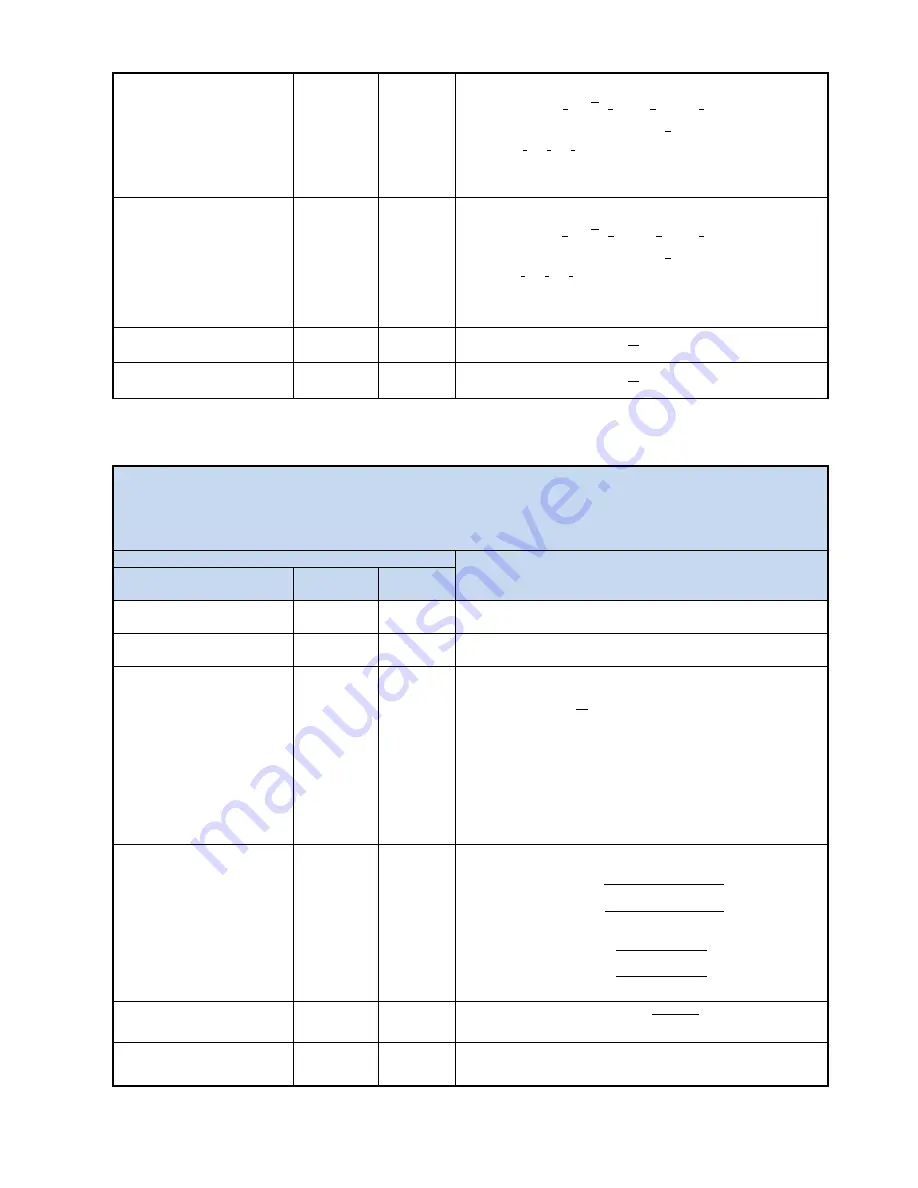
Phase-to-phase voltage
U
CA
U
CA
V
𝑈
𝐶𝐴
= −(𝑈
𝐴𝐵
+ 𝑈
𝐵𝐶
)
Current I
2
(Aron measuring circuits)
I
2
A
𝐼
2
= −(𝐼
1
+ 𝐼
3
)
Total active power
P
tot
W
𝑃
𝑡𝑜𝑡
=
1
𝑀
(∑ 𝑈
𝑖𝐴𝐶
𝐼
𝑖𝐴
𝑀
𝑖=1
+ ∑ 𝑈
𝑖𝐵𝐶
𝐼
𝑖𝐵
𝑀
𝑖=1
)
where:
U
iAC
is a subsequent sample of voltage
U
A-C
U
iBC
is a subsequent sample of voltage
U
B-C
I
iA
is a subsequent sample of current
I
A
I
iB
is a subsequent sample of current
I
B
M
= 2048 for 50 Hz and 60 Hz
Total apparent power
S
e
VA
𝑆
𝑒
= 3𝑈
𝑒
𝐼
𝑒
where:
𝑈
𝑒
= √
𝑈
𝐴𝐵
2
+ 𝑈
𝐵𝐶
2
+ 𝑈
𝐶𝐴
2
9
𝐼
𝑒
= √
𝐼
𝐴
2
+ 𝐼
𝐵
2
+ 𝐼
°𝐶
2
3
Total reactive power (Bu-
deanu and IEEE 1459)
Q
Btot
var
𝑄 = 𝑁 = √𝑆
𝑒
2
− 𝑃
2
Total Budeanu distortion
power
D
Btot
var
𝐷
𝐵𝑡𝑜𝑡
= 0
Summary of Contents for PQM-700
Page 85: ...85 Notes...