Differential Equation Graphing
413
Note:
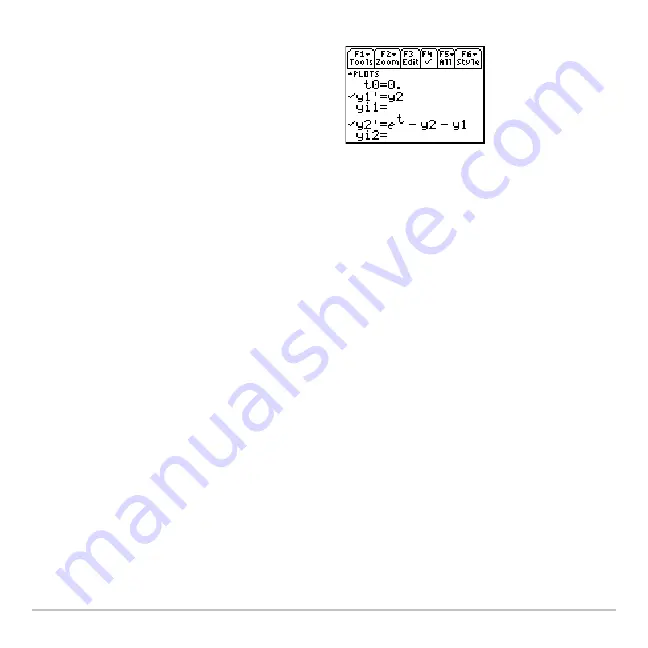
Based on the above substitutions, the y' lines in the Y= Editor represent:
y1' = y'
y2' = y''
etc.
Therefore, this example’s 2nd-order equation is entered on the y2' line.
In a system such as this, the solution to the y1' equation is the solution to the nth-order
equation. You may want to deselect any other equations in the system.
Example of a 2nd-Order Equation
The 2nd-order differential equation y''+y = 0 represents a simple harmonic oscillator.
Transform this into a system of equations for the Y= Editor. Then, graph the solution for
initial conditions y(0) = 0 and y'(0) = 1.
2. On the applicable lines in the Y= Editor,
define the system of equations as:
y1' = y2
y2' = y3
y3' = y4
– up to –
yn ' = your nth-order equation
Summary of Contents for Titanium TI-89
Page 9: ...Getting Started 6 TI 89 Titanium keys Ë Ì Í Ê ...
Page 34: ...Getting Started 31 2 or D 2 B u s i n e s s D B D B Press Result ...
Page 43: ...Getting Started 40 3 0 D B D D B D Press Result ...
Page 44: ...Getting Started 41 D 2 0 0 2 D B Scroll down to October and press Press Result ...
Page 58: ...Getting Started 55 Example Set split screen mode to TOP BOTTOM Press Result 3 B D ...
Page 70: ...Getting Started 67 ...
Page 175: ...Operating the Calculator 172 From the Keyboard ...
Page 456: ...Tables 453 ...
Page 527: ...Data Matrix Editor 524 ...
















































